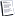 need help from a math wiz!!!
need help from a math wiz!!!
Hello,
Here we go....
Were R is the radius, AC is perpendicular to BO. We are trying to find the area in semicircle ABCD.
------------
First, find the area of the pie ABCO.
R = AO = BO = CO = 1.25"
BD = 0.5"
DO = BO - BD = 1.25" - 0.5" = 0.75"
Angle DOC = inv cos (DO / CO)
Angle DOC = 0.9273 rad or 53.13 degrees.
Since AD = DC, then angle DOC = angle DOA.
Therefore Angle AOC = 2 * angle DOC = 106.26 deg.
A circle has 360 degrees, so the pie ABCO is 106.26 / 360 = 29.52% of the circle.
Area of ABCO pie = pi * R * R * pie's percentage of the circle.
Area of ABCO pie = 1.4489 square inches.
-------------
Next, find area of triangle ADCO.
DC = AD = sqrt (CO * CO - DO * DO)
DC = AD = 1"
Area of ADCO = AC * DO / 2 = 0.75 square inches.
--------------
Area of the semicircle.
Semicircle ABCD = Pie ABCO - Triangle ADCO
Semicircle ABCD = 0.69889 square inches.
- Victor.
[ 23 October 2001: Message edited by: reefburnaby ]
|